Flyby anomaly by light of superluminal

Abstract

 The observer contracts isotropically due to the rotation of the earth, and experiences superluminal light propagation (fly-by anomaly).

What is the Flyby Anomaly?

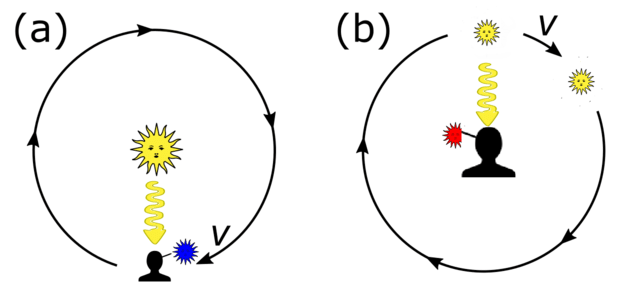
The flyby anomaly is a discrepancy between current scientific models and the actual increase in speed (i.e. increase in kinetic energy) observed during a planetary flyby (usually of Earth) by a spacecraft. In multiple cases, spacecraft have been observed to gain greater speed than scientists had predicted, but thus far no convincing explanation has been found. This anomaly has been observed as shifts in the S-band and X-band Doppler and ranging telemetry. The largest discrepancy noticed during a flyby has been 13 mm/s.[1]

Possible explanations

There have been a number of proposed explanations of the flyby anomaly, including:

・It has been postulated that the Flyby Anomaly is a consequence of the assumption that the speed of light is isotropic in all frames, and invariant in the method used to measure the velocity of the space probes by means of the Doppler Effect.[14] The inconsistent anomalous values measured: positive, null or negative are simply explained relaxing this assumption. During flyby maneuvers the velocity components of the probe in the direction of the observer (Vo) are derived from the relative displacement (df) of the radiofrequency (f) transmitted by the probe, multiplied by the local speed of the light (c') by the Doppler effect: (Vo＝[df／f]c'). According to the Céspedes-Curé hypothesis,[15], the movement through variable gravitational energy density fields produces slight variations of the refractive-index (n') of space, and therefore of the speed of light (c') which leads to unaccounted corrections of the Doppler data that are based on an invariant (c). This leads to incorrect estimates of the speed or energy change in the flyby maneuver on the Earth’s frame of reference.

・Unaccounted transverse Doppler effect—i.e. the red-shift of light source with zero radial and non-zero tangential velocity.[13] However, this cannot explain the similar anomaly in the ranging data.

Explanation by the asymmetric Doppler effect of light

 Since the observer of DSN is isotropically contracted due to the rotation motion (ω R cos θ), the frame to be observed seems to be light of superluminal (W₊) when viewed from the observation reference frame of the speed of light (C).

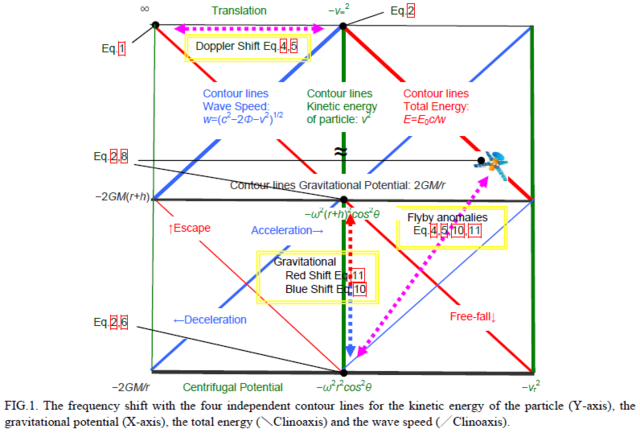
W₊＝√(C²＋[ω R cos θ]²)　　(1).

 The difference in speed of light ( C<W₊ ) due to the contraction of the observer in the DSN appears in the difference in infinity velocity (V∞' < V∞) of the spacecraft.

V∞＝(W₊／C)V∞',

　√(V∞²－V∞'²)／V∞≅ω R cos θ／C　　(2).

 When this observer moves, the observed frequency (f) of the asymmetric Doppler effect of light is,

f＝f₊／(1－V₊ cos α／W₊) 　　(3).

α: Moving angle of the light source seen from the observer = Angle of the observer seen from the moving direction of the light source.

 The secondary Doppler shift (f₊) for the motion does not appear in the reciprocating signal emitted from the moving observer and reflected from the object (reference: [d] in the figure below).

　 However, the flyby anomaly appears in the primary Doppler shift.

⊿f／f₀＝(f－f₀)／f₀＝⊿V∞ cos α／W₊　　(4).

 When α in (Equation 4) (the two directions of declination δi and δo are based on the equator of the earth) is connected to the DSN station at latitude θ in (Equation 2), the following Anderson relational expression is obtained.

⊿V∞／(2 V∞’[cos δ i－cos δo])

＝ω R／C

＝√(V∞²－V∞'²)／(V∞ cos θ)　　(5).

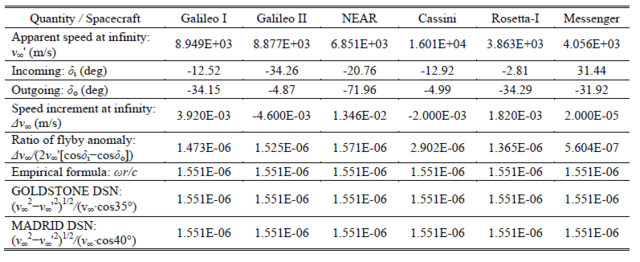
When the orbit of the spacecraft is applied to the hyperbolic trajectory in contact with it, and the asymptotic declination of approach and exit is (δi, δo), this equation (ΔV∞／V∞＝K[cos δi－cos δo]) is expressed. However, the left side is the proportion of anomalies represented by hyperbolic excess velocity. The proportional coefficient K is (K ≈ 3.099 × 10-6), which is expressed as (K = 2ω R / c) using the Earth's rotation angular velocity ω and the equatorial radius R. However, c is the speed of light.

Conclusion

 Mbelek stated that "this anomaly is apparent transverse Doppler effect in special relativity.". However, he casts it into a non-relativistic primary Doppler formula to explain the anomaly. This means that the observer's time is delayed according to the observer's movement, but the phenomenon cannot be explained only by the contraction of space-time in the direction of travel.

Lorenz, Absolute time + Object contracts in the direction of travel.

Einstein, Relative time + Time and space contracts in the direction of travel.

This paper, Relative time + Object contracts in the isotropically.

 The observer's time is delayed, the observer contracts isotropically, the speed of light of other frames increases relative to the observer's frame, and the distance increases, resulting in a flyby anomaly in the Doppler effect and range data. Therefore, the formula for the asymmetric Doppler effect of light changes.

 This result must be reconsidered not only for the time dilation but also for the isotropic contraction of the object.

For mathematical consistency, Lorentz proposed a new time variable, the "local time", called that because it depended on the position of a moving body, following the relation t'=t-vx/c². [p13]. Lorentz considered local time not to be "real"; rather, it represented an ad hoc change of variable.

 Like the flyby anomaly, it is difficult to obtain valid results for a moving observer, and has long relied on an approximation of the invariant speed of light. However, it can be said that the paradigm shift from the absolute stationary frame to the observational reference frame of speed of light is still halfway.

Acknowledgments

 I am grateful for the email response from Dr. Anderson, who was the leader of this fly-by anomaly proposal, and I thank those who discussed these on the web site.